Lines do not exist in our physical world due to the simple fact that their properties will always have a deficiency of a third dimension. Lines are one dimensional and exist only on planar surfaces.
The moment that a line possesses another axis or dimension of measurement, it immediately acquires a degree of width or cross-section thereby dissolving the very existence of the line itself. In our experience of the world, we might have been conditioned occasionally to view the existence of lines in everyday objects. On closer examination, unless they are traces of a moving point without widths lying on a plane, these viewed lines are thinly stretched three-dimensional objects such as a taut string, a flat bar, cracks in between, a long rod, wires, series of objects or people, and other such represented dimensions of a line but never the actual line itself.
Lines, therefore, are only a hypothetical figment of geometry and cannot exist in our three-dimensional world. Nevertheless, it is quite fascinating to note that we often use them to delineate our views of the world and design our aspirations of the world we want to live in.
The accepted mathematical definition of a straight line is the shortest distance between two points on a plane. If there are a point P and another point Q in space, a straight line passing through these two points P and Q could traverse across that space on a plane endlessly. A line, strictly speaking, also has no ends.
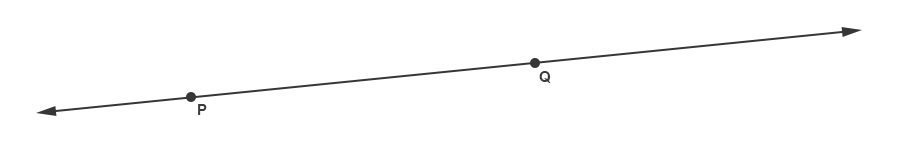
The parody of this mathematical definition of shortest distance is that in reality, the line does not visually perform the distance created by these two points. Even as viewers, we cannot instantly anticipate the value of this distance more so that part of the straight-line attribute is that it can extend infinitely on both ends.
It can even be observed that when we remove this visible line, the tension between the remaining two points creates a much more felt and exaggerated distance.

So it seems that despite attempts of meanings in geometry, true lines consequently are not about distances in as much as how the gap between the two points is.
When a line is drawn on a blank plane, what actually is being constructed are the delineation of spaces and the birth of spaces.
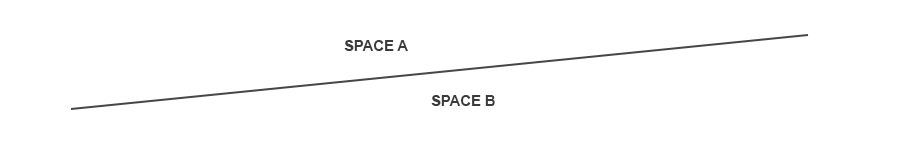
A straight line across a blank plane dissects the plane into two areas like a boundary fence which simultaneously defines both sides of that fence. Where before the existence of that line, the undefined boundaries of that plane prevent us from any reference for it to being a space because there is nothing for it to be identified within the first place. This delineation thereby induces an opposition, a duality of the existence of the same fabric of the plane, but no longer as one element even if these spaces still share the same parts elsewhere. In the example above, the areas in which Space A and Space B occupy are not clearly understood near the ends of the line because technically they are still merged with the same plane. The role of this line that cuts across this plane then becomes crucial in creating this awareness of identities as spaces through references and restrictions. The closer the proximity to the center of this line is, the more defined the identities of the spaces are.

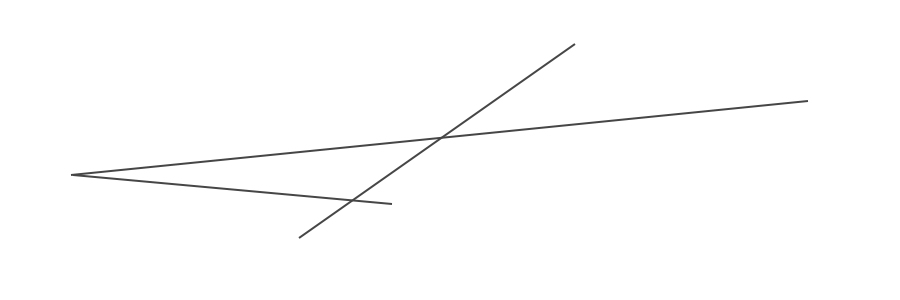
If we were to add another line where they conjoin at their ends to form an angle, the nature of these lines then transforms into active enclosures. Now where space resides outside of the acute angle such as in the example, Space-A is now an open space. But where space is inside this acute angle such as Space B becomes an interior space. Suddenly our references to these spaces become either of the inside or the outside because of additional lines and their placements.
With the introduction of other lines, biases are inadvertently formed.
The further we add biases by introducing more lines on to the plane, the further the plane is divided to create new spaces. Whether space is fully enclosed by lines or open, we become more cognizant of the being or identities of these spaces as they near to where the lines lie. The spaces created in this manner, however, start to take up a unique ability. Against the positive marks of the lines, spaces gradually adopt anonymity. Precipitously they recede within the depths of the background plane where the lines are drawn in. We know that the spaces are there but we strain to make these spaces visible. Where once these spaces start to occupy, they now appear more like cavities or voids as the enclosure made by these lines becomes more complete. The lines appear more forward as the foreground.
What becomes more evident now are the lines themselves orchestrating to deliver outlines. These orchestrations of outlines, in turn, generate a visible configuration of forms. The interesting attribute of these developing forms is that they would naturally represent a familiar shape in the physical world depending on the interpretation of the viewer whether the purpose of the maker was intentional or accidental. Our cognitive reaction to the outline formations is to acknowledge certain patterns and to try piece together these patterns to deduce a comprehensible thought or reason. It is then of natural consequence that the primal medium of a drawing is to trace outlines that eventually represent most natural world explanations.
The lines that continually divide the plane into spaces create perceived enclosures as outlines which then generate familiar forms that graphically induce an idea or message. It is as if these linear forms that eventually cultivates to designs, from sketches to fabrications, are developed through the delineation of spaces using lines in a way similar to the act of cell divisions to create life. Then the process of designs can be concluded that it begins through the delineation of spaces and not a culmination of drawings as it was mostly perceived to be.
Then again lines do not really exist in our physical world because as we have defined earlier, they are one dimensional. If we were to draw a line on paper using pencil and use a microscope to view this pencil mark, we would see that the line made no matter how thinly constructed it would still have some kind of line thickness. Since lines technically do not have widths or thicknesses, the line made onto that paper surface does not exist. The images or ideas that are induced through these forms made from these invisible lines whether representational or for design applications are essentially induced from nothing. The lines that did not exist gave the authority to create for something. In reality, when we refer to these lines as a start to design our world anywhere from tangible products to architecture, we are stimulating from nothing.
Therefore the divisions of planar spaces made by non-existing lines are merely hallucinations that induce our reasoning from the forms they make which in turn we delineate and design our realities with.
Leave a Reply